Triangles: Class 10 Mathematics NCERT Chapter 6
Key Features of NCERT Material for Class 10 Mathematics Chapter 6 – Triangles
In the previous chapter 5, you learned about Arithmetic Progression. In this chapter, you will learn about Triangles.
SIMILAR FIGURES
Two figures having a similar shape however redundant a similar size are called similar figures.
All congruent figures are similar yet all similar figures not necessarily are congruent.
SIMILAR POLYGONS
Two polygons are considered to be similar to one another, if:
(I) their corresponding angles are equivalent, and
(ii) the lengths of their corresponding sides are relative/proportional
Model:
Any two line segments are similar since the length is corresponding
Any two circles are similar since radii are relative/ proportional
Any two squares are similar since corresponding angles are equivalent and lengths are relative/ proportional
Note:
Similar figures are congruent if there is a balanced correspondence between the figures.
∴ From above we find:
Any two triangles are similar if their
(I) Corresponding angles are equivalent
![]()
∠A = ∠P
∠B = ∠Q
∠C = ∠R
(ii) Corresponding sides are relative(proportional)
THALES THEOREM OR BASIC PROPORTIONALITY THEORY
Theorem 1:
State and demonstrate Thales’ Theorem.
Statement:
In the event that a line is constructed parallel aside of a triangle to intersect the other different sides in unmistakable points, the other different sides are isolated in a similar ratio.

From (I), (ii) and (iii),
![]()
Basis FOR SIMILARITY OF TRIANGLES
Two triangles are similar if the accompanying three bases’ of both the triangles are fulfilled:
AAA similarity Criterion. In the event that two triangles are equiangular, at that point they are similar.
Corollary(AA similarity). In the event that two angles of one triangle are separately equivalent to two angles of another triangle, at that point the two triangles are similar.
SSS Similarity Criterion. In the event that the corresponding sides of two triangles are relative, at that point they are similar.
SAS Similarity Criterion. In the event that in two triangles, one set of corresponding sides are relative and the included angles are equivalent, at that point the two triangles are similar.
Similar Triangles based on Similarity Criterion:
The ratio of corresponding sides is equal to Ratio of corresponding edges
The ratio of corresponding sides = Ratio of corresponding medians
The ratio of corresponding sides = Ratio of corresponding heights
The ratio of corresponding sides = Ratio of corresponding point bisector segments.
AREA OF SIMILAR TRIANGLES
Theorem 2.
The ratio of the areas of two similar triangles is equivalent to the square of the ratio of their corresponding sides.
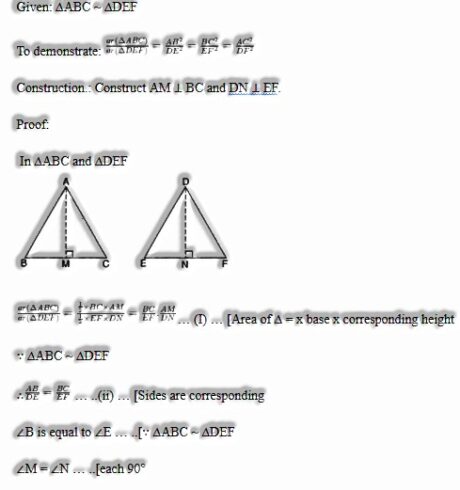

The ratio of areas of two similar triangles = Ratio of squares of corresponding heights
The ratio of areas of two similar triangles is equal to Ratio of squares of corresponding medians
The ratio of areas of two similar triangles is equal to Ratio of squares of corresponding edge bisector segments.
Note:
In the event that the areas of two similar triangles are equivalent, the triangles are congruent.
PYTHAGORAS THEOREM
Theorem 3:
State and demonstrate Pythagoras’ Theorem.
Articulation:
Demonstrate that, in a right-angled triangle, the square of the hypotenuse is equivalent to the total of the squares of the other different sides.
Given: ∆ABC is a right-angled triangle angled at B.
To demonstrate: AB² + BC² = AC²
Const.: Draw BD ⊥ AC
Verification: In ∆s ABC and ADB,
∠A = ∠A … [common
∠ABC = ∠ADB … [each 90°
∴ ∆ABC ~ ∆ADB … [AA Similarity
∴ … [sides are proportional]
⇒ AB² = AC.AD
Presently in ∆ABC and ∆BDC
∠C = ∠C … ..[common]
∠ABC = ∠BDC … .[each 90°]
∴ ∆ABC ~ ∆BDC … ..[AA similarity]
∴ … ..[sides are proportional]
BC² = AC.DC … (ii)
On including (I) and (ii), we get
AB² + BC² = ACAD + AC.DC
⇒ AB² + BC² is equal to AC.(AD + DC)
AB² + BC² = AC.AC
∴AB² + BC² = AC²
Opposite OF PYTHAGORAS THEOREM
Theorem 4:
State and demonstrate the contrast of Pythagoras’ Theorem.
Statement:
Demonstrate that, in a triangle, if the square of one side is equivalent to the total of the squares of the other different sides, at that point the edge inverse to the principal side is a right edge.
Given: In ∆ABC, AB² + BC² = AC²
To demonstrate: ∠ABC = 90°
Const.: Draw a privilege calculated ∆DEF in which DE = AB and EF = BC
Evidence: In ∆ABC,
AB² + BC² is equal to AC² … (I) [given]
In rt. ∆DEF
DE² + EF² = DF² … [by pythagoras theorem]
AB² + BC² = DF² … ..(ii) … [DE = AB, EF = BC]
From (I) and (ii), we get
AC² = DF²
⇒ AC = DF
Presently, DE = AB … [by cont]
EF = BC … [by cont]
DF = AC … .[proved above]
∴ ∆DEF ≅ ∆ABC … [sss congruence]
∴ ∠DEF = ∠ABC … ..[CPCT]
∠DEF = 90° … [by cont]
∴ ∠ABC = 90°
Results based on Pythagoras’ Theorem:
(I) Result on obtuse Triangles.
In the event that ∆ABC is an obtuse triangle, obtuse-angled at B,
In the event that AD ⊥ CB, at that point
AC² is equal to AB² + BC² + 2 BC.BD
(ii) Result on Acute Triangles.
When ∆ABC is an acute-angled triangle, acute-angled at B, and AD ⊥ BC, at that point AC² = AB² + BC² – 2 BD.BC.

